A Two-State Retrial Queueing Model with Feedback having Two Identical Parallel Servers

Neelam Singla; Harwinder Kaur

doi:10.17485/IJST/v14i11.1167

Article

A Two-State Retrial Queueing Model with Feedback having Two Identical Parallel Servers

VIEWS 1269
PDF 284

Indian Journal of Science and Technology

DOI: 10.17485/IJST/v14i11.1167

Year: 2021, Volume: 14, Issue: 11, Pages: 915-931

Original Article

A Two-State Retrial Queueing Model with Feedback having Two Identical Parallel Servers

Neelam Singla¹, Harwinder Kaur^2*

¹Assistant Professor, Department of Statistics, Punjabi University, Patiala, 147002, India
²Research Scholar, Department of Statistics, Punjabi University, Patiala, 147002, India. Tel.:+99465311134

*Corresponding Author
Tel: +91-9465311134
Email: [email protected]

Received Date:17 July 2020, Accepted Date:26 February 2021, Published Date:09 April 2021

This work is licensed under a Creative Commons Attribution 4.0 International License.

Abstract

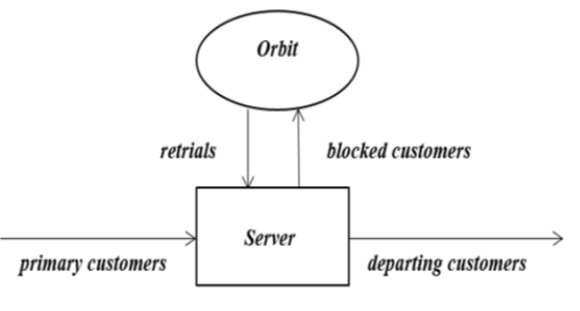
Objective: In this study we consider a two-state retrial queueing system with feedback having two identical parallel servers. Transient state probabilities for exact number of arrivals and departures from the system will be obtained when both, one or none of the servers is busy. Numerical and graphical solutions will also be obtained. Methods: The difference-differential equations governing the system are solved recursively, Laplace transform is then used to obtain the transient state probabilities for exact number of arrivals and departures from the system. Findings: Time dependent probabilities are obtained when both, one and none of the servers is busy. Numerical and Graphical solutions are also obtained using MATLAB programming. Novelty: In past research, models considered arrivals and departures from the orbit whereas in present model arrivals and departures from the system are studied along with the concept of feedback. Applications: This type of model is implemented in computer systems. Mathematics Subject Classification: 60K25, 90B22, 60M20

Keywords: Arrivals; Departures; Queueing; Retrial; Feedback

References

Cohen JW. Basic problems of telephone traffic theory and the influence of repeated calls. Philips Telecommunication Review . 1957;18(2):49–100.
Yang T, Templeton JGC. A survey on retrial queues. Queueing Systems. 1987;2:201–233. Available from: https://dx.doi.org/10.1007/bf01158899
Artalejo JR, Gomez-Corral A. A Computational Approach. In: Retrial queueing systems (1). (pp. XIII-318) 1999. https://doi.org/10.1007/978-3-540-78725-9
Artalejo J, Falin G. Standard and retrial queueing systems: a comparative analysis. Revista Matemática Complutense. 2002;15(1):101–129. Available from: https://dx.doi.org/10.5209/rev_rema.2002.v15.n1.16950
Finch PD. Cyclic Queues with Feedback. Journal of the Royal Statistical Society: Series B (Methodological). 1959;21(1):153–157. Available from: https://dx.doi.org/10.1111/j.2517-6161.1959.tb00323.x
Takacs L. 1963. Available from: https://doi.org/10.1002/j.1538-7305.1963.tb00510.x
Lee YW. The M/G/1 feedback retrial queue with two types of customers. Bulletin of the Korean Mathematical Society. 2005;42(4):875–887.
Mokaddis G, Metwally S, Zaki B. A feedback retrial queuing system with starting failures and single vacation. Tamkang Journal of Science and Engineering. 2007;10(3):183–192.
Kumar BK, Vijayalakshmi G, Krishnamoorthy A, Basha SS. A single server feedback retrial queue with collisions. Computers & Operations Research. 2010;37(7):1247–1255. Available from: https://dx.doi.org/10.1016/j.cor.2009.04.019
Shekhar C, Jain M. Finite Queueing Model with Multitask Servers and Blocking. American Journal of Operational Research. 2013;3(2A):17–25.
Yang DY, Ke JC, Wu CH. The multi-server retrial system with Bernoulli feedback and starting failures. International Journal of Computer Mathematics. 2015;92(5):954–969.
Nobel R. Retrial queueing models in discrete time: a short survey of some late arrival models. Annals of Operations Research. 2016;247(1):37–63. Available from: https://dx.doi.org/10.1007/s10479-015-1904-7
Pegden CD, Rosenshine M. Some New Results for theM/M/1 Queue. Management Science. 1982;28(7):821–828. Available from: https://dx.doi.org/10.1287/mnsc.28.7.821
Indra R. Transient analysis of markovian queueing model with bernoulli schedule and multiple working vacations. Int J Comput Appl. 2011;20:43–48.
Singla N, Kalra S. Performance Analysis of a Two-State Queueing Model with Retrials Journal of Rajasthan . Academy of Physical Sciences. 2018;17(1 & 2):81–100.
Singla N, Kalra S. A Two-State Multiserver Queueing System with Retrials. International Journal of Open Problems in Computer Science & Mathematics. 2019;12(3):62–75.
Falin G, Templeton JG. Retrial queues. (Vol. 75) CRC Press. 1997.
Sztrik J. Basic Queueing Theory. 1986.

Copyright

© 2021 Singla & Kaur.This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited. Published By Indian Society for Education and Environment (iSee).