Comparative Study of Crank-Nicolson and Modified Crank-Nicolson Numerical methods to solve linear Partial Differential Equations

Tejaskumar C Sharma; Shreekant P Pathak; Gargi Trivedi

doi:10.17485/IJST/v17i10.1776

Article

Comparative Study of Crank-Nicolson and Modified Crank-Nicolson Numerical methods to solve linear Partial Differential Equations

VIEWS 119
PDF 35

Indian Journal of Science and Technology

DOI: 10.17485/IJST/v17i10.1776

Year: 2024, Volume: 17, Issue: 10, Pages: 924-931

Original Article

Comparative Study of Crank-Nicolson and Modified Crank-Nicolson Numerical methods to solve linear Partial Differential Equations

Tejaskumar C Sharma^1*, Shreekant P Pathak², Gargi Trivedi³

¹Research Scholar, Department of Mathematics, N V Patel College of Pure & Applied Sciences, The Charutar Vidyamandal University, Vallabh Vidyanagar, Anand, Gujarat, India
²Assistant Professor, Department of Mathematics, N V Patel College of Pure & Applied Sciences, The Charutar Vidyamandal University, Vallabh Vidyanagar, Anand, Gujarat, India
³Department of Applied Mathematics, Faculty of Technology and Engineering, The Maharaja Sayajirao University of Baroda, Vadodara, Gujarat, India

*Corresponding Author
Email: [email protected]

Received Date:17 July 2023, Accepted Date:30 January 2024, Published Date:27 February 2024

This work is licensed under a Creative Commons Attribution 4.0 International License.

Abstract

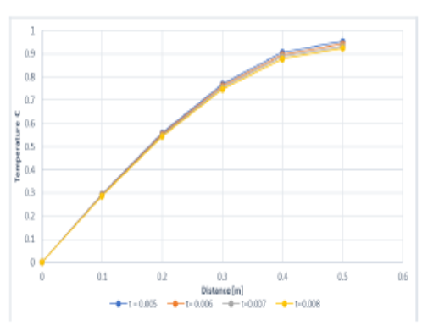
Objectives: This paper aims to address the limitations of the Crank-Nicolson Finite Difference method and propose an improved version called the modified Crank-Nicolson method. Methods: Utilized implicit discretization in time and space, with parameters k = 0.001, h = 0.1, and γ = 0.1. Conducted extensive testing on various partial differential equations. Findings: Results, displayed in Table 1, showcase the method's stability and accuracy. Comparative analysis in Table 2 demonstrates the Modified Crank-Nicolson method consistently outperforming the traditional approach, reaffirming its superiority in accuracy. Novelty: The modified Crank-Nicolson method offers a significant enhancement to the traditional Crank-Nicolson finite difference method, making it a valuable tool for effectively solving partial differential equations.

Keywords: CrankNicolson Method, Modified CrankNicolson Method, Finite Difference, Partial Differential Equations, Parabolic Equations, Python Software

References

Abhulimen CE, Omowo BJ. Modified Crank-Nicolson method for solving one dimensional parabolic equation. International Journal of Scientific Research. 2019;15(6):60–66. Available from: https://doi.org/10.9790/5728-1506036066
Gorbova TV, Pimenov VG, Solodushkin SI. Crank–Nicolson Numerical Algorithm for Nonlinear Partial Differential Equation with Heredity and Its Program Implementation. In: P, S, K, A, V, V., eds. Mathematical Analysis With Applications. (Vol. 318, pp. 33-43) Springer International Publishing. 2020.
Kafle J, Bagale LP, JKCD. Numerical Solution of Parabolic Partial Differential Equation by Using Finite Difference Method. Journal of Nepal Physical Society. 2020;6(2):57–65. Available from: https://doi.org/10.3126/jnphyssoc.v6i2.34858
Trivedi GJ, Sanghvi R, Shah VS, Sharma JP. On solution of non-instantaneous impulsive Hilfer fractional integro-differential evolution system. Mathematica Applicanda. 2023;51(1):3–20. Available from: https://www.researchgate.net/publication/364293591_On_Solution_of_Non-instantaneous_Impulsive_Hilfer_Fractional_Integro-differential_Evolution_System
Trivedi GJ, Sanghvi R. Medical Image Fusion Using CNN with Automated Pooling. Indian Journal Of Science And Technology. 2022;15(42):2267–2274. Available from: https://doi.org/10.17485/IJST/v15i42.1812
Ajeel OA, Gaftan AM. Using Crank-Nicolson Numerical Method to solve Heat-Diffusion Problem. Tikrit Journal of Pure Science. 2023;28(3):101–104. Available from: https://www.iasj.net/iasj/download/2127585509e30adf
Tarmizi T, Safitri E, Munzir S, Ramli M. On the numerical solutions of a one-dimensional heat equation: Spectral and Crank Nicolson method. In AIP Conference Proceedings . 2020;2268. Available from: https://doi.org/10.1063/5.0017131
Erfanifar R, Sayevand K, Ghanbari N, Esmaeili H. A modified Chebyshev ϑ‐weighted Crank–Nicolson method for analyzing fractional sub‐diffusion equations. Numerical Methods for Partial Differential Equations. 2021;37(1):614–625. Available from: https://doi.org/10.1002/num.22543
Costa PJ. Select Ideas in Partial Differential Equations. Springer International Publishing. 2021. Available from: https://link.springer.com/book/10.1007/978-3-031-02434-4
Wick T. Numerical Methods for Partial Differential Equations. Available from: https://doi.org/10.15488/11709
Salsa S, Verzini G. Partial Differential Equations in Action. Partial differential equations in action: from modelling to theory. 2022;147. Available from: https://link.springer.com/book/10.1007/978-3-031-21853-8
Yang WY, Cao W, Chung T, Morris J. Applied Numerical Methods Using MATLAB®. Wiley. 2005. Available from: https://doi.org/10.1002/9781119626879
Koroche KA. Numerical solution for one dimensional linear types of parabolic partial differential equation and application to heat equation. Mathematics and Computer Science. 2020;5(4). Available from: https://doi.org/10.11648/j.mcs.20200504.12
Sathyapriya S, Hamsavarthini G, Meenakshi M, Tanushree E. A Study on Crank Nicolson Method for Solving Parabolic Partial Differential Equations. 2021. Available from: https://ijrti.org/papers/IJSDR2107009.pdf
Sharma T, Pathak SS, Trivedi GJ, Sanghvi RR. Flow Modelling in Porous Medium Applying Numerical Techniques: A Comparative Analysis. Recent Research Reviews Journal. 2023;2(2):288–304. Available from: https://www.researchgate.net/publication/376115762_Flow_Modelling_in_Porous_Medium_Applying_Numerical_Techniques_A_Comparative_Analysis
Omowo BJ, Abhulimen CE. Crank-Nicolson and Modified Crank-Nicolson Scheme for One Dimensional Parabolic Equation. International Journal of Applied Mathematics and Theoretical Physics. 2020;6(3):35–40. Available from: https://doi.org/10.11648/j.ijamtp.20200603.11
Omowo BJ, Abhulimen CE. On the stability of Modified Crank-Nicolson method for Parabolic Partial differential equations. International Journal of Mathematical Sciences and Optimization: Theory and Applications. 2020;6(2):862–873. Available from: https://www.ajol.info/index.php/ijmso/article/view/253350

Copyright

© 2024 Sharma et al. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited. Published By Indian Society for Education and Environment (iSee)