A Novel Chaotic System with Wide Spectrum, its Synchronization, Circuit Design and Application to Secure Communication

Piyush Pratap Singh

doi:10.17485/IJST/v14i28.1035

Article

A Novel Chaotic System with Wide Spectrum, its Synchronization, Circuit Design and Application to Secure Communication

VIEWS 1349
PDF 309

Indian Journal of Science and Technology

DOI: 10.17485/IJST/v14i28.1035

Year: 2021, Volume: 14, Issue: 28, Pages: 2351-2367

Original Article

A Novel Chaotic System with Wide Spectrum, its Synchronization, Circuit Design and Application to Secure Communication

Piyush Pratap Singh^1*

¹Department of Electrical Engineering, NIT Meghalaya, Laitumkhrah, Shillong, 793003, Meghalaya, India

*Corresponding Author
Email: [email protected]

Received Date:08 June 2021, Accepted Date:20 July 2021, Published Date:24 August 2021

This work is licensed under a Creative Commons Attribution 4.0 International License.

Abstract

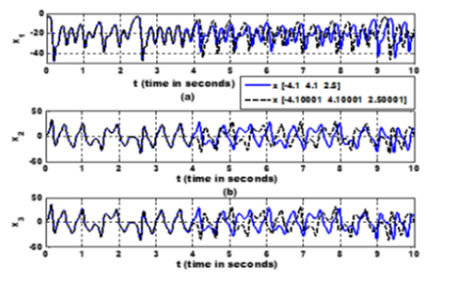
Objective: To investigate a novel chaotic system with unique features, its synchronization using nonlinear active control, analog circuit design and application to secure communication. Methods/Analysis: Dynamical tools such as dissipative analysis, instability of equilibrium points, sensitivity to initial conditions, 0-1 test, recurrence plot, Poincare map, Lyapunov exponents, Lyapunov dimension, frequency spectrum and basin of attraction. Synchronization is achieved using modified nonlinear active control technique and analog circuit design, implementation is done in NI Multisim platform. MATLAB and Multisim results are presented to meet the adequate verification of theoretical approach. Findings: A three-dimensional chaotic system with only two nonlinear terms, three parameters and a total of eight terms are proposed. The proposed system has three saddle focus type equilibria. The proposed system is topologically different from Lorenz’s and Rossler’s, Lu’s, Chen’s, and Liu’s families. Such dynamic systems are very few in the literature as per authors best knowledge. The system has basin of chaotic attractors for which first Lyapunov exponent ranges between 2.5 to 3. Frequency spectrum and large positive Lyapunov exponent result comparatively large bandwidth of the proposed systems against some well-known chaotic systems. Chaos, periodic and stable behaviors are obtained by altering the system parameters. Novelty/Application: The proposed three-dimensional chaotic system has significant chaotic behavior and broader spectrum than the six chaotic systems like Lorenz, Rossler, Lu, Chen, BG and Liu systems. Unlike the conventional active control approach, the proposed nonlinear active control does not result decoupled error dynamics. The system has significantly large bandwidth which is helpful in the masking of message signals and enhances the security of transmitted signals during communication.

Keywords: Analog circuit design; Chaos; Chaotic system; Chaosbased communication; Chaos synchronization; Nonlinear active control

References

Volkovskii AR, Tsimring L, Rulkov SNF, LL. and Langmore I. Spread spectrum communication system with chaotic frequency modulation. An Interdisciplinary Journal of Nonlinear Science. 2005;15. Available from: https://doi.org/10.1063/1.1942327
Nuñez-Perez JC, Adeyemi VA, Sandoval-Ibarra Y, Pérez-Pinal FJ, Tlelo-Cuautle E. FPGA Realization of Spherical Chaotic System with Application in Image Transmission. Mathematical Problems in Engineering. 2021;2021:Article ID 5532106. Available from: https://doi.org/10.1155/2021/5532106
Marek B. Chaos Predictability in a Chemical Reactor. International Journal of Bifurcation and Chaos. 2020;30(11):2050221. Available from: https://doi.org/10.1142/S0218127420502211
Saheb P, Mainul H, Pijush P, Pati NC, Pal N, Joydev C. Cooperation delay induced chaos in an ecological system. An Interdisciplinary Journal of Nonlinear Science. 2020;30(8):83124. Available from: https://doi.org/10.1063/5.0012880
Sahin ME, Taskiran ZGC, HG, SEH. Application and Modeling of a Novel 4D Memristive Chaotic System for Communication Systems. Circuits, Systems, and Signal Processing. 2020;39:3320–3349. Available from: https://doi.org/10.1007/s00034-019-01332-6
Singh PP, Roy BK. Inter network synchronization of complex dynamical networks by using smooth proportional integral SMC technique. European Physics Journal Special Topics. 2020;229:861–876. Available from: https://doi.org/10.1140/epjst/e2020-900149-3
Edward NL. Deterministic nonperiodic flow. Journal of the Atmospheric Sciences. 1963;20:130–141. Available from: https://doi.org/10.1175/1520-0469(1963)020<0130:DNF>2.0.CO;2
Rossler OE. An equation for continuous chaos. Physics Letters A. 1976;57(5):90101–90109. Available from: https://doi.org/10.1016/0375-9601(76)90101-8
Chen CH, Sheu LJ, Chen HK, Chen JH, Wang HC, Chao YC, et al. A novel hyper-chaotic system and its synchronization. Nonlinear Analysis: Real World Applications. 2009;10(4):2088–2096. Available from: https://doi.org/10.1016/j.nonrwa.2008.03.015
Julien CS. Chaos and Time-Series Analysis. London. Oxford University Press. 2003.
Chongxin L, Tao LT, Ling L, Kai L. A novel chaotic attractor. Chaos, Solitons and Fractals. 2004;22(5):1031–1038. Available from: https://doi.org/10.1016/j.chaos.2007.04.025
Jinhu L, Guanrong C. A novel chaotic attractor coined. International Journal of Bifurcation and Chaos. 2002;12(3):659–661. Available from: https://doi.org/10.1142/S0218127402004620
Wenbo L, Guanrong C. A novel chaotic system and its generation. International Journal of Bifurcation Chaos. 2003;13(1):261–267. Available from: https://doi.org/10.1142/S0218127403006509
Singh PP, BKR. A novel chaotic system without equilibria, with parachute and thumb shapes of Poincare map and its projective synchronization. European Physics Journal Special Topics. 2020;229:1265–1278. Available from: https://doi.org/10.1140/epjst/e2020-900259-0
Guoyuan Q, Guanrong C, Yuhui Z. On a novel asymmetric chaotic system. Chaos Solitons and Fractals. 2008;37:409–423. Available from: https://doi.org/10.1016/j.chaos.2006.09.012
Ihsan P, Yılmaz U. A novel chaotic attractor from general Lorenz system family and its electronic experimental implementation. Turkish Journal of Electrical Engineering and Computer Sciences. 2010;18(2).
Singh PP, Roy BK. Comparative performances of synchronization between different classes of chaotic systems using three control techniques. Annual Reviews in Control. 2018;45(3):152–165. Available from: https://doi.org/10.1016/j.arcontrol.2018.03.003
Ihsan P, Yılmaz U. A novel 3D chaotic system with golden proportion equilibria: Analysis and electronic circuit realization. Computers and Electrical Engineering. 2012;38:1777–1784. Available from: https://doi.org/10.1016/j.compeleceng.2012.08.007
Vaidyanathan S, Ihsan P. Analysis, control, synchronization, and circuit design of a novel chaotic system. Mathematical and Computer Modelling. 2012;55:1904–1915. Available from: https://doi.org/10.1016/j.mcm.2011.11.048
Singh PP, Roy BK. Memristor-based novel complex-valued chaotic system and its projective synchronization using nonlinear active control technique. European Physics Journal Special Topics. 2019;228:2197–2214. Available from: https://doi.org/10.1140/epjst/e2019-900036-5
Abooee A, Yaghini-Bonabi HA, Jahed-Motlagh MR. Analysis and circuitry realization of a novel three-dimensional chaotic system. Communications in Nonlinear Science and Numerical Simulation. 2013;18:1235–1245. Available from: https://doi.org/10.1016/j.cnsns.2012.08.036
Yongjian L. Circuit implementation and finite-time synchronization of the 4D Rabinovich hyperchaotic system. Nonlinear Dynamics. 2012;67:89–96. Available from: https://doi.org/10.1007/s11071-011-9960-2
Ahmed GR, Ahmed MS, ESAL. MOS realization of the double-scroll-like chaotic equation. IEEE Transactions on Circuits and Systems-I: Fundamental Theory and Applications. 2003;50(2):285–288. Available from: https://doi.org/10.1109/TCSI.2002.808217
Louis MP, Thomas LC. Synchronization of chaotic systems. Chaos: An Interdisciplinary Journal of Nonlinear Science. 2015;25:97611. Available from: https://doi.org/10.1063/1.4917383
Singh PP, Singh JP, Roy BK. Synchronization and anti-synchronization of Lu and Bhalekar-Gejji chaotic systems using nonlinear active control. Chaos, Solitons and Fractals. 2014;69:31–39. Available from: https://doi.org/10.1016/j.chaos.2014.09.005
Vaidyanathan S. Adaptive design of controller and synchronizer for Lu-Xiao chaotic system with unknown parameters. International Journal on Computer Science and Information Technology. 2013;5:197–210. Available from: https://doi.org/10.5121/IJCSIT.2013.5116
Singh PP, Singh KM, Roy BK. Chaos control in biological system using recursive backstepping sliding mode control. European Physics Journal Special Topics. 2018;227:731–746. Available from: https://doi.org/10.1140/epjst/e2018-800023-6
Junchan Z, Jun-An L. Using sampled-data feedback control and linear feedback synchronization in a novel hyperchaotic system. Chaos, Solitons and Fractals. 2008;35:376–382. Available from: https://doi.org/10.1016/j.chaos.2006.05.035
Hongmei G, Shouming Z. Synchronization criteria of time-delay feedback control system with sector bounded nonlinearity. Applied Mathematics and Computation. 2009;191:550–557. Available from: https://doi.org/10.1016/j.amc.2007.02.154
Singh PP, Roy BK. Microscopic chaos control of chemical reactor system using nonlinear active plus proportional integral sliding mode control technique. European Physics Journal Special Topics. 2019;228:169–184. Available from: https://doi.org/10.1140/epjst/e2019-800115-4
Faqiang W, Chongxin L. Synchronization of unified chaotic system based on passive control. Physics D: Applied Physics. 2007;225:55–60. Available from: https://doi.org/10.1016/j.physd.2006.09.038
Yu-Ping T, Xinghuo Y. Stabilizing unstable periodic orbits of chaotic systems via an optimal principle. Journal of Franklin Institute. 2000;337:47–55. Available from: https://doi.org/10.1016/S0016-0032(00)00047-8
Jae-Hun K, Chang-Woo P, Euntai K, Mignon P. Fuzzy adaptive synchronization of uncertain chaotic systems. Physics Letters A. 2004;334:295–305. Available from: https://doi.org/10.1016/j.physleta.2004.11.033
Coelho LDS, Bernert DLDA. PID control design for chaotic synchronization using a Tribes optimization approach. Chaos, Solitons and Fractals. 2009;42:634–640. Available from: https://doi.org/10.1016/j.chaos.2009.01.032
Bernardini D, Grzegorz L. An overview of 0-1 test for chaos. Journal of the Brazilian Society of Mechanical Sciences and Engineering. 2016;38(5):1433–1450. Available from: https://doi.org/10.1007/s40430-015-0453-y
Sabarathinam S, TK. Transient chaos in a globally coupled system of nearly conservative Hamiltonian Duffing oscillators. Chaos, Solitons and Fractals. 2015;73:129–140. Available from: https://doi.org/10.1016/j.chaos.2015.01.004
Norbert M, Romano MC, Thiel M, Kurths J. Recurrence Plots for the Analysis of Complex Systems. Physics Reports. 2007;438(5-6):237–329. Available from: https://doi.org/10.1016/j.physrep.2006.11.001
Iftikhar A, Chunlai M, Fuchen Z. A novel chaotic attractor with quadratic exponential nonlinear term from Chen’s attractor. International Journal of Analysis and Applications. 2014;5(1):27–32. Available from: http://etamaths.com/index.php/ijaa/article/view/197
Fa-Qiang W, Chong-Xin L. Hyperchaos evolved from the Liu chaotic system. Chinese Physics. 2006;15(5):963–966. Available from: https://doi.org/10.1088/1009-1963/15/5/016
Bruce AR, Stéphane M, Pier M, Erik B. Nonlinear characterization of a Rossler system under periodic closed-loop control via time-frequency and bispectral analysis. Mechanical Systems and Signal Processing. 2018;99:567–585. Available from: https://doi.org/10.1016/j.ymssp.2017.06.001

Copyright

© 2021 Singh. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited. Published By Indian Society for Education and Environment (iSee)