Indian Journal of Science and Technology

Abstract

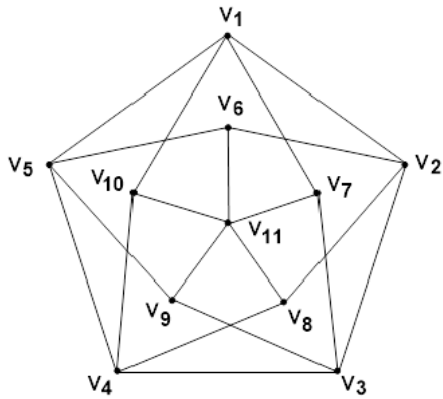
Objectives: The minimum degree energy concept is applied to pebbling graphs. This study establishes a connection between the minimum degree energy of graphs and the minimum degree energy of pebbling graphs by applying the lowest degree energy of pebbling notion to twenty standard graphs. Methods: The minimum degree energy of pebbling graph with the matrix whose was calculated using . The characteristic polynomial of the minimum degree, is must be found from the matrix. Next, the eigen values of the matrix were calculated using and the sum of all the eigen values gives the minimum degree energy. The lower and upper bounds for the minimum degree energy of graphs are established along with the algorithm for computing the minimum degree energy of graphs. Findings: The lower and upper bounds were found for the minimum degree energy of pebbling graphs. For twenty standard graphs and pebbling graphs, the minimum degree energy values were calculated, and their relation was tabulated. Novelty: Pebbling graphs were subjected to the minimal energy idea and a relationship was found between the minimum energy of pebbling graphs and the minimum energy of graphs in general.

Keywords: Energy, Minimum degree energy, Grotzsch graph, Pebbling graph, Data mining