Stationary solutions, critical mass, Tadpole orbits in the circular restricted three-body problem with the more massive primary as an oblate spheroid

A Arantza Jency; Ram Krishan Sharma; Gagandeep Singh

doi:10.17485/IJST/v13i39.1396

Article

Stationary solutions, critical mass, Tadpole orbits in the circular restricted three-body problem with the more massive primary as an oblate spheroid

VIEWS 2346
PDF 319

Indian Journal of Science and Technology

DOI: 10.17485/IJST/v13i39.1396

Year: 2020, Volume: 13, Issue: 39, Pages: 4168-4188

Original Article

Stationary solutions, critical mass, Tadpole orbits in the circular restricted three-body problem with the more massive primary as an oblate spheroid

A Arantza Jency^1*, Ram Krishan Sharma¹, Gagandeep Singh¹

¹Department of Aerospace Engineering, Karunya Institute of Technology and Sciences, Coimbatore, 641114, Tamil Nadu, India. Tel.: +91-9566406224

*Corresponding Author
Tel: +91-9566406224
Email: [email protected]

Received Date:13 August 2020, Accepted Date:21 October 2020, Published Date:09 November 2020

This work is licensed under a Creative Commons Attribution 4.0 International License.

Abstract

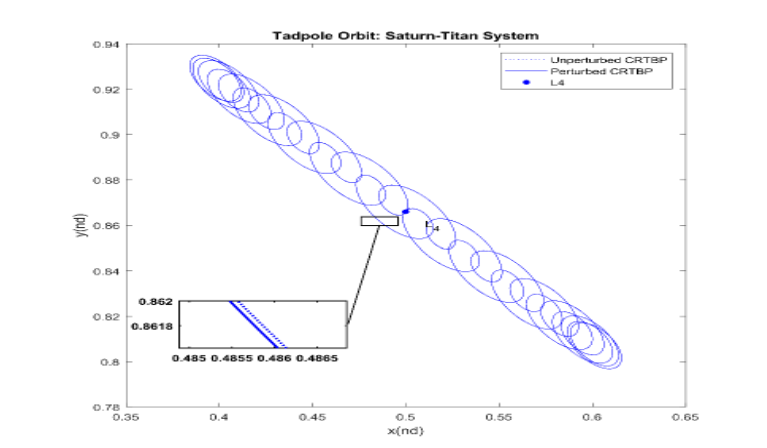
Background: The location and stability of the equilibrium points are studied for the Planar Circular Restricted Three-Body Problem where the more massive primary is an oblate spheroid. Methods: The mean motion of the equations of motion is formulated from the secular perturbations as derived by(1) and used in(2–4). The singularities of the equations of motion are found for locating the equilibrium points. Their stability is analysed using the linearized variational equations of motion at the equilibrium points. Findings: As the effect of oblateness in the mean motion expression increases, the location and stability of the equilibrium points are affected by the oblateness of the more massive primary. It is interesting to note that all the three collinear points move towards the more massive primary with oblateness. It is a new result. Among the shifts in the locations of the five equilibrium points, the y–location of the triangular equilibrium points relocate the most. It is very interesting to note that the eccentricities (e) of the orbits around L1 and L3 increase, while it decreases around L2 with the addition of oblateness with the new mean motion. The decrease in e is significant in Saturn-Mimas system from 0.95036 to 0.87558. Similarly, the value of the critical mass ratio mc, which sets the limit for the linear stability of the triangular points, further reduces significantly from 0:285: : :A1 to 0:365: : :A1 with the new mean motion. The mean motion sz in the z-direction increases significantly with the new mean motion from 9A1/4 to 9A1/2.

Keywords: Circular Restricted Three-Body Problem (CRTBP); oblateness; mean motion; equilibrium points; critical mass ratio; tadpole orbits

References

Sharma RK, Sellamuthu H, Jency AA. Perturbed Trojan dynamics in the solar system. In: AAS AIAA Astrodynamics Specialist Conference . p. 20–704.
Johnson A, Sharma RK. Locations of Lagrangian points and periodic orbits around triangular points in the photo gravitational elliptic restricted three-body problem with oblateness. International Journal of Advanced Astronomy. 2019;7:25–38. Available from: https://doi.org/10.14419/ijaa.v7i2.29377
Jency AA, Sharma RK. Location and stability of the triangular Lagrange points in photo-gravitational elliptic restricted three body problem with the more massive primary as an oblate spheroid. International Journal of Advanced Astronomy. 2019;7(2). Available from: https://dx.doi.org/10.14419/ijaa.v7i2.29814
Arohan R, Sharma RK. Periodic orbits in the planar restricted photo-gravitational problem when the smaller primary is an oblate spheroid. Indian Journal of Science and Technology. 2020;13(16):1630–1640. Available from: https://doi.org/10.17485/IJST/v13i16.401
Sharma RK. Perturbations of Lagrangian points in the restricted three-body problem. Indian Journal of Pure and Applied Mathematics. 1975;6:1099–1102.
Sharma RK, Rao PVS. Collinear equilibria and their characteristic exponents in the restricted three-body problem when the primaries are oblate spheroids. Celestial Mechanics. 1975;12(2):189–201. Available from: https://dx.doi.org/10.1007/bf01230211
McCuskey SW. Introduction to Celestial Mechanics. Addison-Wesley Publishing Company. 1963.
Rao PVS, Sharma RK. A note on the stability of the triangular points of equilibrium in the restricted three-body problem. Astronomy and Astrophysics. 1975;43:381–383. Available from: http://adsabs.harvard.edu/abs/1975A%26A....43..381S
Sharma RK. Study of Periodic Orbits in Restricted Problem taking the Bigger Primary an Oblate Spheroid. Ph.D Thesis, University of Delhi. thesis
Sharma RK, Rao PVS. Stationary solutions and their characteristic exponents in the restricted three-body problem when the more massive primary is an oblate spheroid. Celestial Mechanics. 1976;13(2):137–149. Available from: https://dx.doi.org/10.1007/bf01232721
Sharma RK, Rao PVS. A case of commensurability induced by oblateness. Celestial Mechanics. 1978;18(2):185–194. Available from: https://dx.doi.org/10.1007/bf01228715
Sharma RK, Rao PVS. Effect of oblateness on triangular solutions at critical mass. Astrophysics and Space Science. 1979;60(2):247–250. Available from: https://dx.doi.org/10.1007/bf00644329
Sharma RK. Periodic orbits of second kind in the restricted three-body problem when the more massive primary is an oblate spheroid. Astrophys. And Space Science. 1981;76:255–258. Available from: https://doi.org/10.1007/
Sharma RK. On linear stability of triangular libration points of the photogravitational three-body problem when the more massive primary is an oblate spheroid. In: Fricke W, GT., eds. Sun and Planetary System. (pp. 435-436) Dordrecht, Holland. D. Reidel Publishing Co. 1982.
Sharma RK, Rao PVS. On finite periodic orbits around the equilateral solutions of the planar restricted three-body problem. In: Bhatnagar KB., ed. International workshop on Space Dynamics and Celestial mechanics. D. Reidel Publishing Co. p. 71–85.
Sharma RK. The linear stability of libration points of the photogravitational restricted three-body problem when the smaller primary is an oblate spheroid. Astrophys. Space science. 1987;135:271–281. Available from: https://doi.org/10.1007/BF00641562
Rao PVS, Sharma RK. Oblateness effect on finite periodic orbits at L4. In: 39th Congress of the International Astronautical Federation. 300.
Sharma RK. The periodic orbits of the second kind in terms of Giacaglia’s variables with oblateness. Earth, Moon and Planets. 1989;45:312–318. Available from: https://ui.adsabs.harvard.edu/link_gateway/1989EM&P...45..213S/doi:10.1007/BF00057744
Sharma RK. Periodic orbits of third kind in the restricted three-body problem with oblateness. Astrophys. And Space Science. 1990;166:211–218. Available from: https://doi.org/10.1007/BF01094894
Rao PVS, Sharma RK. Effect of oblateness on the non-linear stability of L 4 in the restricted three-body problem. Celestial Mechanics and Dynamical Astronomy. 1997;65(3):291–312. Available from: https://dx.doi.org/10.1007/bf00053510
Markellos VV, Papadakis KE, Perdios EA. Non-linear stability zones around triangular equilibria in the plane circular restricted three-body problem with oblateness. Astrophysics and Space Science. 1996;245(1):157–164. Available from: https://dx.doi.org/10.1007/bf00637811
Jain S, Kumar A, Bhatnagar KB. Periodic orbits around the collinear liberation points in the restricted three body problem when the smaller primary is a triaxial rigid body: Sun-Earth case. Bull. Astr. Soc. India. 2006;34:211–223. Available from: https://ui.adsabs.harvard.edu/#abs/2006BASI...34..211J/abstract
Shankaran S, Sharma JP, Ishwar B. Equilibrium points in the generalised photogravitational non-planar restricted three body problem. International Journal of Engineering, Science and Technology. 2011;3(2):63–67. Available from: https://dx.doi.org/10.4314/ijest.v3i2.68133
Safiya Beevi A, Sharma RK. Oblateness effect of Saturn on periodic orbits in the Saturn-Titan restricted three-body problem. Astrophysics and Space Science. 2012;340(2):245–261. Available from: https://dx.doi.org/10.1007/s10509-012-1052-3
Arredondo JA, Guo J, Stoica C, Tamayo C. On the restricted three body problem with oblate primaries. Astrophysics and Space Science. 2012;341(2):315–322. Available from: https://dx.doi.org/10.1007/s10509-012-1085-7
Abouelmagd EI, Asiri HM, Sharaf MA. The effect of oblateness in the perturbed restricted three-body problem. Meccanica. 2013;48(10):2479–2490. Available from: https://dx.doi.org/10.1007/s11012-013-9762-3
Tiwary RD, Kushvah BS. Computation of halo orbits in the photogravitational Sun-Earth system with oblateness. Astrophysics and Space Science. 2015;357(1):1–16. Available from: https://dx.doi.org/10.1007/s10509-015-2243-5
Pathak N, Thomas VO. Analysis of Effect of Oblateness of Smaller Primary on the Evolution of Periodic Orbits. International Journal of Astronomy and Astrophysics. 2016;06(04):440–463. Available from: https://dx.doi.org/10.4236/ijaa.2016.64036
Chidambararaj P, Sharma RK. Halo Orbits around Sun-Earth L1 in Photogravitational Restricted Three-Body Problem with Oblateness of Smaller Primary. International Journal of Astronomy and Astrophysics. 2016;06(03):293–311. Available from: https://dx.doi.org/10.4236/ijaa.2016.63025
Ansari AA, Alam M. Dynamics in the circular restricted three body problem with perturbations. International Journal of Advanced Astronomy. 2017;5(1):19. Available from: https://dx.doi.org/10.14419/ijaa.v5i1.7102
Beevi AS, Sharma RK. Effect of Oblateness of the More Massive Primary on Periodic Orbits in the Restricted Three-Body Problem. Advances in Astrophysics. 2017;2:12–24. Available from: https://dx.doi.org/10.22606/adap.2017.21003
Pathak N, Thomas VO, Abouelmagd EI. The perturbed photogravitational restricted three-body problem: analysis of resonant periodic orbits. Discrete and continuous dynamical systems: Series S. 2019;12:849–875. Available from: https://doi.org/10.3934/dcdss.2019057
Szebehely V. Theory Of Orbits. New York. Academic Press. 1967.
Danby JMA. Fundamentals of Celestial Mechanics (2). VA, USA. Willmann-Bell, Inc. 1988.
Murray CD, Dermott SF. Solar System Dynamics. Cambridge. Cambridge University Press. 1999.
Rabe E. Determination and survey of periodic Trojan orbits in the restricted problem of three bodies. The Astronomical Journal. 1961;66(9):500. Available from: https://dx.doi.org/10.1086/108451
Singh J, Ishwar B. Stability of triangular points in the generalised photogravitational restricted three body problem. Astronomical Society of India. 1999;27:415–424. Available from: http://adsabs.harvard.edu/abs/1999BASI...27..415S

Copyright

© 2020 Arantza Jency et al.This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited. Published By Indian Society for Education and Environment (iSee).