Vertex k-Prime Labeling of Theta Graphs

S Teresa Arockiamary; G Vijayalakshmi

doi:10.17485/IJST/v16i26.1278

Article

VIEWS 585
PDF 112

Indian Journal of Science and Technology

DOI: 10.17485/IJST/v16i26.1278

Year: 2023, Volume: 16, Issue: 26, Pages: 2008-2015

Original Article

Vertex k-Prime Labeling of Theta Graphs

S Teresa Arockiamary¹, G Vijayalakshmi^1*

¹Department of Mathematics, Stella Maris College (Autonomous), Affiliated to University of Madras, Chennai, India

*Corresponding Author
Email: [email protected]

Received Date:26 May 2023, Accepted Date:18 June 2023, Published Date:11 July 2023

This work is licensed under a Creative Commons Attribution 4.0 International License.

Abstract

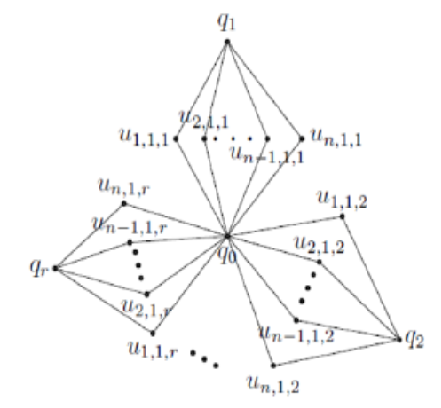
Objectives: To analyse some theta related graphs that admit vertex k-prime labeling for each positive integer k. Methods: In this study, vertices of the graphs are assigned with k, k+1,…,k+|V|-1 such that each pair of labels of adjacent vertices are relatively prime. Justifications for the proof are given. Findings: We examine the theta related graphs such as generalised theta graphs, uniform theta graphs, centralised uniform theta graphs for m = 1 are vertex k-prime. In addition, we introduce another structure of theta graph known as centralised generalised theta graph and show that vertex k-prime labeling exists for the graph. Novelty: Vertex k-prime labeling is a new variant of prime labeling and theta families of graphs exhibiting the labeling is a new finding. Another structure of theta graph known as centralised generalised theta graph is introduced and proved that vertex k-prime labeling exists for the graph.

Keywords: Vertex k-Prime Labeling; Generalised Theta Graphs; Uniform Theta Graphs; Centralised Uniform Theta Graphs; Centralised Generalised Theta Graph

References

Gallian J. A Dynamic Survey of Graph Labeling. The Electronic Journal of Combinatorics. 2022. Available from: https://www.combinatorics.org/files/Surveys/ds6/ds6v25-2022.pdf
Tout A, Dabboucy AN, Howalla K. Prime labeling of graphs. National Academy Science Letters. 1982;11. Available from: https://www.springer.com/journal/40009
Arockiamary T, Vijayalakshmi S, G. Vertex k-prime labeling of cyclic snakes. Communications in Mathematics and Applications. 2023;14(1):9–20. Available from: https://www.rgnpublications.com/journals/index.php/cma/article/view/1989
Arockiamary T, Vijayalakshmi S, G. Vertex k-prime labeling on graphs. European Chemical Bulletin. 2023;12:9627–9633. Available from: https://doi.org/10.48047/ecb/2023.12.si4.864
Rajan B, Rajasingh I, Venugopal P. Metric dimension of uniform and quasi-uniform theta graphs. Journal of Mathematics and Computer Science. 2011;2(1):37–46. Available from: http://www.compmath-journal.org/dnload/-BHARATI-RAJAN-INDRA-RAJASINGH-and-VENUGOPAL-P-/CMJV02I01P0037.pdf
Putra RW, Susanti Y. On total edge irregularity strength of centralized uniform theta graphs. AKCE International Journal of Graphs and Combinatorics. 2018;15(1):7–13. Available from: https://doi.org/10.1016/j.akcej.2018.02.002

Copyright

© 2023 Arockiamary & Vijayalakshmi. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited. Published By Indian Society for Education and Environment (iSee)