Wave Propagation in a Homogeneous Poroelastic Layer Bounded Between Transversely Isotropic Poroelastic Half-space and an in-homogeneous Elastic Half-space

C Nageswaranath; V Sravana Kumar; K Elangovan

doi:10.17485/IJST/v16i20.2322

Article

Wave Propagation in a Homogeneous Poroelastic Layer Bounded Between Transversely Isotropic Poroelastic Half-space and an in-homogeneous Elastic Half-space

VIEWS 494
PDF 107

Indian Journal of Science and Technology

DOI: 10.17485/IJST/v16i20.2322

Year: 2023, Volume: 16, Issue: 20, Pages: 1527-1537

Original Article

Wave Propagation in a Homogeneous Poroelastic Layer Bounded Between Transversely Isotropic Poroelastic Half-space and an in-homogeneous Elastic Half-space

C Nageswaranath^1*, V Sravana Kumar², K Elangovan³

¹Department of Mathematics, BVRIT HYDERABAD College of Engineering for Women, 500090, Telangana, Hyderabad
²Department of Mathematics, Sree Rama Engineering College, Tirupathi, 517507, AndhraPradesh, India
³Mathematics Section, FEAT, Annamalai University, Chidambaram, 608002, Tamilnadu, India

*Corresponding Author
Email: [email protected]

Received Date:25 December 2022, Accepted Date:08 April 2023, Published Date:26 May 2023

This work is licensed under a Creative Commons Attribution 4.0 International License.

Abstract

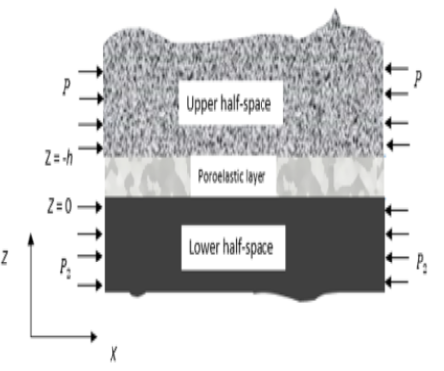
Objectives: The present work investigates the propagation of love waves in an isotropic poroelastic layer that is bounded by an inhomogeneous orthotropic half-space and a transversely isotropic elastic half-space. The transversely isotropic poroelastic layer and elastic half-space are taken as initially stressed. Methods: Using Biot’s theory, the equations of motion for poroelastic layers are derived. The dispersion equation for phase velocity has been derived analytically. Findings: The effect of initial stresses of both elastic half-space and layer is observed. Also, the dispersion equation is derived for poroelastic layer and elastic layer as particular cases. Numerical work has been carried out and results are presented graphically. Novelty: The propagation of Love waves at the boundary between two elastic substances has received a lot of attention. Present study deals with three solid structures, a layer bounded between two half-spaces.

Keywords: Poroelastic; Transversely isotropic; Phase velocity; Wave number; Initially stressed

References

Chinta N, Syed AS, Modem R, Mangipudi VR. A Study on Propagation of Waves in a Transversely Isotropic Poroelastic Layer Bounded between Two Viscous Liquids. Open Journal of Acoustics. 2019;9(1):1–12. Available from: https://doi.org/10.4236/oja.2019.91001
Kumar; RAJ22RviafltlpcssbafJM, Nageswaranath C, Kavitha K, Shah SA. Radial vibrations in a fluid-loaded two layered poroelastic cylindrical shell surrounded by a fluid. API Conference Proceedings. 2020;2246(1). Available from: https://doi.org/10.1063/5.0015000
Othman MIA, Zidan MEM, Mohamed IEA. Effect of magnetic field and gravity on two-temperature thermomicrostretch elastic medium under dual-phase lag model. Indian Journal of Physics. 2020;94(1):69–79. Available from: https://doi.org/10.1007/s12648-019-01444-4
Li YC, Feng SJ, Chen HX, Chen ZL, Zhang DM, Huang HW. Dynamic response of a stratified transversely isotropic half-space with a poroelastic interlayer due to a buried moving source. Applied Mathematical Modelling. 2020;82:45–71. Available from: https://doi.org/10.1016/j.apm.2020.01.036
Gupta AK, Mukhopadhyay AK, Patra P, Kundu S, Patra S, Kundu. Love wave in porous layer under initial stress over heterogeneous elastic half-space under gravity and initial stress. . Geofísica internacional- Ciudad de México. 2021;60(3):193–210. Available from: https://doi.org/10.22201/igeof.00167169p.2021.60.3.1917
Mahmoodian M, Eskandari-Ghadi M, Nikkhoo A. Body waves propagation in a fluid-saturated transversely isotropic poroelastic solid with a potential method. Scientia Iranica. 2021;28(3):1132–1151. Available from: https://doi.org/10.24200/sci.2020.54428.3748
Wang Y, Lin G, Li Z. Dynamic Response of Anisotropic Multilayered Road Structures Induced by Moving Loads Based on a Novel Spectral Element Method. Buildings. 2022;12(9):1354. Available from: https:// doi.org/10.3390/buildings12091354
Bharti U, Vaishnav PK. Anti-Plane Wave Propagation in the Functionally Graded Hybrid Structure under an External Impulsive Force: A Green’s Function Approach. . Mathematical Problems in Engineering. 2022;2022(2697447). Available from: https://doi.org/10.1155/2022/2697447
Biot MA. Mechanics of Deformation and Acoustic Propagation in Porous Media. Journal of Applied Physics. 1962;33(4):1482–1498. Available from: https://doi.org/10.1063/1.1728759
Biot MA, Drucker DC. Mechanics of Incremental Deformation. Journal of Applied Mechanics. 1965;32(4):957–958.
Biot MA. Theory of Propagation of Elastic Waves in a Fluid‐Saturated Porous Solid. I. Low‐Frequency Range. The Journal of the Acoustical Society of America. 1956;28(2):168–178. Available from: https://doi.org/10.1121/1.1908239
Kakar R. Dispersion of love wave in an isotropic layer sandwiched between orthotropic and prestressed inhomogeneous half-spaces. Latin American Journal of solids and structures. 2015;12:1934–1949. Available from: https://doi.org/10.1590/1679-78251918
Kakar R, Kakar S. Love-Type Surface Wave in an Isotropic Layer Bounded between Orthotropic and Heterogeneous Half-Spaces under Initial Stresses. International Journal of Geomechanics. 2016;17(3):04016083. Available from: https://doi.org/10.1061/(ASCE)GM.1943-5622.0000765

Copyright

© 2023 Nageswaranath et al. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited. Published By Indian Society for Education and Environment (iSee)