Indian Journal of Science and Technology

Abstract

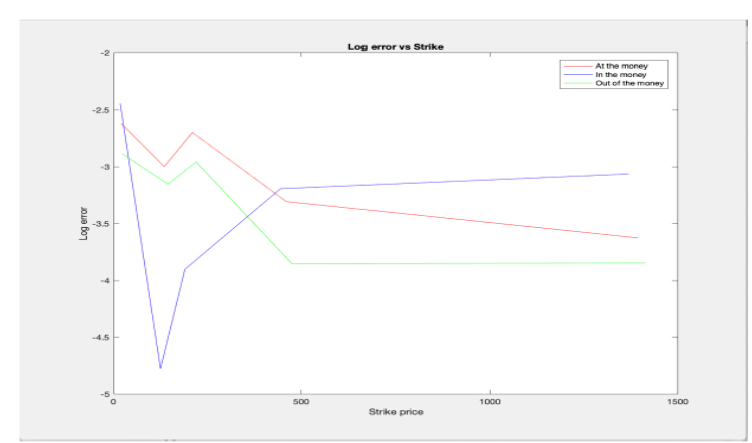
Introduction: Implied volatility is one of the most commonly used estimator to predict future stock market behaviours. Accordingly, millions of option prices are used to compute the implied volatility in the stock market frequently. Traditionally, this is calculated by inverting Black-Scholes option prices with iterative numerical methods but this associates high computational cost. Objectives: This research was mainly focused on implementing and validating an explicit closed-form formula for the implied volatility by using market observed call option prices. Methods: In order to obtain the explicit formula, the Taylor series of the Black-Schole call option price with respect to the volatility around a pre-determined initial value was obtained using the operator calculus and the Faa’ di Bruno’s formula. Taylor series of the implied volatility was acquired using the Lagrange inversion theorem. Here, all the coefficients were explicitly determined using known functions and constants. Findings: The developed equation was tested using real time market call option prices with the corresponding market listed implied volatilities for options were used as the initial values. Numerical examples illustrate a significant accuracy of the formula. Novelty : It is a closed form formula where the coefficients are explicitly determined and free of numerical iterations making it suitable for industrial implementations and adaptation.

Keywords: Implied Volatility; Taylor Series; Faa’ di Bruno’s Formula; Operator Calculus; Lagrange Inversion Theorem