Indian Journal of Science and Technology

Abstract

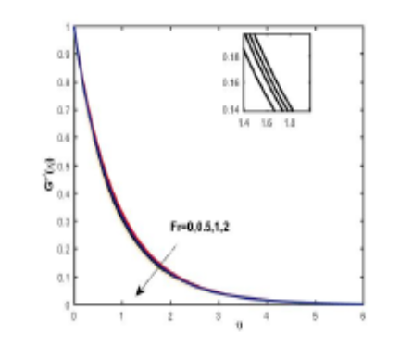
Objective: The present study analyses the heat transfer properties of a Casson fluid moving under the influence of an inclined magnetic field through an exponentially stretched surface in a porous medium using the Darcy-Forchheimer law. Non-Newtonian fluid behavior is described by using the Casson fluid model. Thermophoresis as well as Brownian motion effects on heat transmission and concentration of nanoparticle are considered. Method: With similarity transformations; nonlinear (PDE) partial differential equation has been changed to (ODEs) ordinary differential equations. By using bvp4c programme in the Matlab software, the nonlinear PDE are numerically solved. Findings: The impacts of dimensionless factors on the flow, concentration of nanoparticle and heat transfer were studied. Graphs were plotted and analyzed in order to explore how different dimensionless factors affected velocity, temperature concentration profiles. Novelty: The combination of magnetic fields, nanofluids, and the Darcy-Forchheimer model is an interdisciplinary approach. Future researchers in fields like fluid dynamics, magneto hydrodynamics, materials science, and applied mathematics could benefit from this research work. It bridges multiple disciplines and contributes to the ongoing efforts to make energy-related processes more efficient and sustainable. The findings demonstrate that the porous medium is accountable for both inflation in the thermal boundary layer thickness and a decrease in the thickness of the momentum boundary layer. For increasing the permeability of the medium, conductive heat transfer predominates. The improvement of heat and mass transport is made possible by all these elements.

Keywords: Casson fluid, Heat transmission, Nonlinear PDE., Darcy-Forchheimer law, Porous medium