Indian Journal of Science and Technology

Abstract

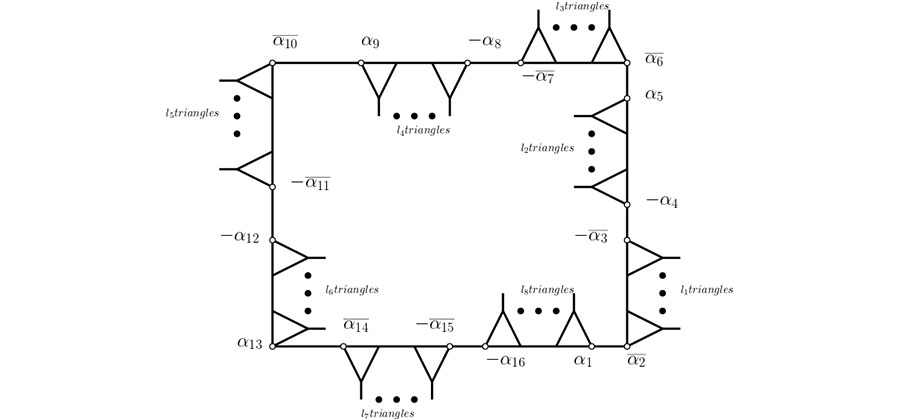
Background/Objectives: Modular group or PSL(2,Z) is a well-known group of the non-singular square matrices of order two by two with unit determinant. An Action of this group on real quadratic fields is represented by coset diagrams consisting of closed paths known as circuits. For a particular value of n , one or more than one circuits combine to form an orbit. The length of a circuit identifies all the circuits of an orbit. The main objective of this study is to explore all the circuits of length eight and their corresponding orbits. Methods: Some circuit equivalent properties, the group theoretical approach, along with the statistical methodology, is adopted to classify the orbits containing circuits of length eight. We also use already discovered results related to circuits of lengths two, four, and six to formulate the basis for our new results of length eight. Findings: We have discovered all the equivalence classes for the circuits of length eight, which are twenty-one in number. For a particular reduced number α, circuits of length eight can have all four; α,−α, its algebraic conjugateα¯ and − α¯ , either in one circuit or (α) G = (−α) G with (−α) G = (−α¯) G or (α) G = (α¯) G with (−α) G = (−α) G or (α) G = (−α) G with (−α) G = (α) G , depending upon the equivalent class of the circuit. Moreover, we have introduced reduced positions and G-midway and discovered that for any reduced numbers αi starting from α1 , reduced positions have a recurring pattern α2,−α3,−α4,α5, and so on. Applications: PSL(2, Z) orbits are entirely classified and drawn, along with the cyclically equivalent circuits of length eight.

Keywords: Modular group; coset diagram; reduced numbers; G-midway; reduced positions