On the Solution of Blasius Boundary Layer Equations of Prandtl-Eyring Fluid Flow Past a Stretching Sheet

Hiteshkumar G Bariya; Manisha P Patel

doi:10.17485/IJST/v17i12.2055

Article

On the Solution of Blasius Boundary Layer Equations of Prandtl-Eyring Fluid Flow Past a Stretching Sheet

VIEWS 97
PDF 34

Indian Journal of Science and Technology

DOI: 10.17485/IJST/v17i12.2055

Year: 2024, Volume: 17, Issue: 12, Pages: 1237-1244

Original Article

On the Solution of Blasius Boundary Layer Equations of Prandtl-Eyring Fluid Flow Past a Stretching Sheet

Hiteshkumar G Bariya¹, Manisha P Patel^2*

¹Research Scholar, Gujarat Technological University, Ahmedabad, Gujarat, India
²Assistant Professor, Sarvajanik College of Engineering & Technology, Surat, Gujarat, India

*Corresponding Author
Email: [email protected]

Received Date:12 August 2023, Accepted Date:09 February 2024, Published Date:20 March 2024

This work is licensed under a Creative Commons Attribution 4.0 International License.

Abstract

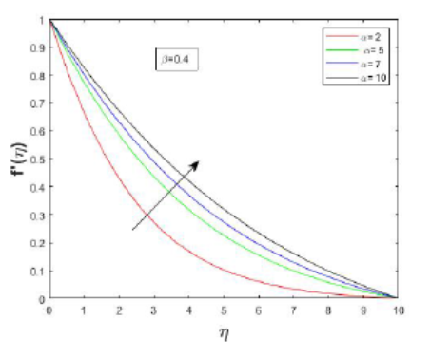
Objective: This paper investigates velocity profile for two-dimensional, incompressible, laminar forced convection flow of the fluid model for Prandtl-Eyring fluid past a stretching sheet in the presence of fluid parameters. Methods: The governing partial differential equation for the flow was transformed into non-linear ordinary differential equation by using the deductive one parameter group theoretic method and numerical solution of non-linear ordinary differential equation (ODE) is solved by MATLAB bvp4c solver. Findings: The solution of velocity profile obtained as a function of parameter and . The effect of the fluid parameter was discussed graphically. Novelty: The main goal of this article is to analyze boundary layer flow of Prandtl-Eyring fluid over a stretching surface. The conservation equations of mass, momentum are converted into non-linear ordinary differential equations along with boundary conditions using deductive one parameter group theoretic method and solved by MATLAB ODE solver. Comparisons with previously published works are made, and results show a high level of agreement. This type of research is applicable to extrusion, paper production, fiber glass production, hot rolling, condensation process, crystal growing, polymer sheets etc.

Keywords: Boundary layer, laminar flow, Deductive one parameter Group theoretic method, Absolute invariant, Stretching Sheet, Prandtl-Eyring fluid

References

Sakiadis BC. Boundary‐layer behavior on continuous solid surfaces: I. Boundary‐layer equations for two‐dimensional and axisymmetric flow. AIChE Journal. 1961;7(1):26–28. Available from: https://doi.org/10.1002/aic.690070108
Sakiadis BC. Boundary‐layer behavior on continuous solid surfaces: II. The boundary layer on a continuous flat surface. AIChE Journal. 1961;7(2):221–225. Available from: https://doi.org/10.1002/aic.690070211
Sakiadis BC. Boundary‐layer behavior on continuous solid surfaces: III. The boundary layer on a continuous cylindrical surface. AIChE Journal. 1961;7(3):467–472. Available from: https://doi.org/10.1002/aic.690070325
Darji RM, Timol MG. Deductive group invariance analysis of boundary layer equations of a special non-Newtonian fluid over a stretching sheet. International Journal of Mathematics and Computer Science. 2012;2(2):54–58. Available from: https://scholar.google.co.in/citations?view_op=view_citation&hl=en&user=ha1tXFsAAAAJ&citation_for_view=ha1tXFsAAAAJ:2osOgNQ5qMEC
Patel M, Timol MG. On the MHD boundary layer flow past a moving continuous flat surface. International Journal of Applied Mathematics and Mechanics. 2013;9(2):1–8. Available from: https://scholar.google.com/citations?view_op=view_citation&hl=en&user=zD-gfC4AAAAJ&cstart=20&pagesize=80&citation_for_view=zD-gfC4AAAAJ:eQOLeE2rZwMC
Rajput GR, Prasad JK, Timol MG. Similarity flow solution of MHD boundary layer model for non-Newtonian power-law fluids over a continuous moving surface. General Mathematics Notes. 2014;24(2):97–102. Available from: http://emis.icm.edu.pl/journals/GMN/yahoo_site_admin/assets/docs/10_GMN-6212-V24N2.334222403.pdf
Darji RM, Timol MG. On invariance analysis of MHD boundary layer equations for non-Newtonian Williamson fluids. International Journal of Applied Mathematics and Mechanics. 2014;1(4):10–19. Available from: https://scholar.google.com/citations?view_op=view_citation&hl=en&user=ha1tXFsAAAAJ&citation_for_view=ha1tXFsAAAAJ:Y0pCki6q_DkC
Shukla H, Patel J, Surati HC, Patel M, Timol MG. Similarity solution of forced convection flow of Powell-Eyring & Prandtl-Eyring fluids by group-Theoretic method. Mathematical Journal of Interdisciplinary Sciences. 2017;5(2):151–165. Available from: https://doi.org/10.15415/mjis.2017.52012
Umar M, Akhtar R, Sabir Z, Wahab HA, Zhiyu Z, Imran A, et al. Numerical Treatment for the Three-Dimensional Eyring-Powell Fluid Flow over a Stretching Sheet with Velocity Slip and Activation Energy. Advances in Mathematical Physics. 2019;2019:1–12. Available from: https://doi.org/10.1155/2019/9860471
Jafar AB, Shafie S, Ullah I, Safdar R, Jamshed W, Pasha AA, et al. Mixed convection flow of an electrically conducting viscoelastic fluid past a vertical nonlinearly stretching sheet. Scientific Reports. 2022;12(1):1–13. Available from: https://doi.org/10.1038/s41598-022-18761-0
Abbas W, Megahed AM, Ibrahim MA, Said AAM. Ohmic dissipation impact on flow of Casson-Williamson fluid over a slippery surface through a porous medium. Indian Journal of Physics. 2023;97:4277–4283. Available from: https://doi.org/10.1007/s12648-023-02754-4
Narsingani LS, Patel VV, Panchal J. Analyze the Flow Behavior for MHD Power-Law Fluids. Indian Journal Of Science And Technology. 2023;16(1):47–55. Available from: https://doi.org/10.17485/IJST/v16i1.1383
Hayat AU, Ullah I, Khan H, Alam MM, Hassan AM, Khan H. Numerical analysis of radiative hybrid nanomaterials flow across a permeable curved surface with inertial and Joule heating characteristics. Heliyon. 2023;9(11):1–12. Available from: https://doi.org/10.1016/j.heliyon.2023.e21452
Ullah I, Alghamdi M, Xia WFF, Shah SI, Khan H. Activation energy effect on the magnetized-nanofluid flow in a rotating system considering the exponential heat source. International Communications in Heat and Mass Transfer. 2021;128. Available from: https://doi.org/10.1016/j.icheatmasstransfer.2021.105578
Ullah S, Ullah I, Ali A. Soret and Dufour effects on dissipative Jeffrey nanofluid flow over a curved surface with nonlinear slip, activation energy and entropy generation. Waves in Random and Complex Media. 2023. Available from: https://doi.org/10.1080/17455030.2022.2164380
Li YMM, Ullah I, Alam MM, Khan H, Aziz A. Lorentz force and Darcy-Forchheimer effects on the convective flow of non-Newtonian fluid with chemical aspects. Waves in Random and Complex Media. 2022. Available from: https://doi.org/10.1080/17455030.2022.2063984
Hayat AU, Ullah I, Khan H, Weera W, Galal AM. Numerical Simulation of Entropy Optimization in Radiative Hybrid Nanofluid Flow in a Variable Features Darcy–Forchheimer Curved Surface. Symmetry. 2022;14(10):1–17. Available from: https://doi.org/10.3390/sym14102057
Ullah I, Shukat S, Albakri A, Khan H, Galal AM, Jamshed W. Thermal performance of aqueous alumina–titania hybrid nanomaterials dispersed in rotating channel. International Journal of Modern Physics B. 2023;37(24). Available from: https://doi.org/10.1142/S0217979223502375
Pathan A, Timol MG. Similarity solution of MHD boundary layer flow of Prandtl-Eyring fluids. International Journal of Engineering Research & Technology. 2015;3(23):1–5. Available from: https://www.ijert.org/similarity-solution-of-mhd-boundary-layer-flow-of-prandtl-eyring-fluids
Hayat T, Bibi S, Alsaadi F, Rafiq M. Peristaltic Transport of Prandtl-Eyring Liquid in a Convectively Heated Curved Channel. PLOS ONE. 2016;11(6):1–19. Available from: https://doi.org/10.1371/journal.pone.0156995
Hussain A, Malik MY, Awais M, Salahuddin T, Bilal S. Computational and physical aspects of MHD Prandtl-Eyring fluid flow analysis over a stretching sheet. Neural Computing and Applications. 2019;31(S1):425–433. Available from: https://doi.org/10.1007/s00521-017-3017-5
Rehman KU, Awais M, Hussain A, Kousar N, Malik MY. Mathematical analysis on MHD Prandtl-Eyring nanofluid new mass flux conditions. Mathematical Methods in the Applied Sciences. 2019;42(1):24–38. Available from: https://doi.org/10.1002/mma.5319
Qayyum S, Hayat T, Kanwal M, Alsaedi A, Khan MI. Transportation of entropy optimization in radiated chemically dissipative flow of Prandtl–Eyring nanofluid with activation energy. Computer Methods and Programs in Biomedicine. 2020;184:105130. Available from: https://doi.org/10.1016/j.cmpb.2019.105130
Hussain A, Malik MY, Khan M, Salahuddin T. Application of generalized Fourier heat conduction law on MHD viscoinelastic fluid flow over stretching surface. International Journal of Numerical Methods for Heat & Fluid Flow. 2020;30(6):3481–3496. Available from: https://doi.org/10.1108/HFF-02-2019-0161
Khan I, Hussain A, Malik MY, Mukhtar S. On magnetohydrodynamics Prandtl fluid flow in the presence of stratification and heat generation. Physica A: Statistical Mechanics and its Applications. 2020;540:123008. Available from: https://doi.org/10.1016/j.physa.2019.123008
Rehman A, Salleh Z. Analytical Investigation of Magnetic Field on Unsteady Boundary Layer Stagnation Point Flow of Water-Based Graphene Oxide-Water and Graphene Oxide-Ethylene Glycol Nanofluid over a Stretching Surface. Mathematical Problems in Engineering. 2021;2021:1–8. Available from: https://doi.org/10.1155/2021/8897111
Patil AB, Humane PP, Patil VS, Rajput GR. MHD Prandtl nanofluid flow due to convectively heated stretching sheet below the control of chemical reaction with thermal radiation. International Journal of Ambient Energy. 2022;43(1):4310–4322. Available from: https://doi.org/10.1080/01430750.2021.1888803
Ullah Z, Ullah I, Zaman G, Khan H, Muhammad T. Mathematical modeling and thermodynamics of Prandtl–Eyring fluid with radiation effect: a numerical approach. Scientific Reports. 2021;11(1):1–11. Available from: https://doi.org/10.1038/s41598-021-01463-4
Munjam SR, Gangadhar K, Seshadri R, Rajeswar M. Novel technique MDDIM solutions of MHD flow and radiative Prandtl-Eyring fluid over a stretching sheet with convective heating. International Journal of Ambient Energy. 2022;43(1):4850–4859. Available from: https://doi.org/10.1080/01430750.2021.1922498
Duraihem FZ, Khan AU, Saleem S, Shawana. Mathematical Analysis of Transverse Wall-Shearing Motion via Cross Flow of Nanofluid. Lubricants. 2023;11(3):1–15. Available from: https://doi.org/10.3390/lubricants11030138
Qureshi MA. A case study of MHD driven Prandtl-Eyring hybrid nanofluid flow over a stretching sheet with thermal jump conditions. Case Studies in Thermal Engineering. 2021;28:1–18. Available from: https://doi.org/10.1016/j.csite.2021.101581
Morgan AJA. The reduction by one of the number of independent variables in some systems of partial differential equations. The Quarterly Journal of Mathematics. 1952;3(1):250–259. Available from: https://doi.org/10.1093/qmath/3.1.250
Moran MJ, Gaggioli RA. Reduction of the Number of Variables in Systems of Partial Differential Equations, with Auxiliary Conditions. SIAM Journal on Applied Mathematics. 1968;16(1):202–215. Available from: https://doi.org/10.1137/0116018

Copyright

© 2024 Bariya & Patel. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited. Published By Indian Society for Education and Environment (iSee)