Runge-Kutta Method Based on the Linear Combination of Arithmetic Mean, Geometric Mean, and Centroidal Mean: A Numerical Solution for the Hybrid Fuzzy Differential Equation

P Evangelin Diana Rajakumari; R Gethsi Sharmila

doi:10.17485/IJST/v17i18.75

Article

Runge-Kutta Method Based on the Linear Combination of Arithmetic Mean, Geometric Mean, and Centroidal Mean: A Numerical Solution for the Hybrid Fuzzy Differential Equation

VIEWS 202
PDF 47

Indian Journal of Science and Technology

DOI: 10.17485/IJST/v17i18.75

Year: 2024, Volume: 17, Issue: 18, Pages: 1860-1867

Original Article

Runge-Kutta Method Based on the Linear Combination of Arithmetic Mean, Geometric Mean, and Centroidal Mean: A Numerical Solution for the Hybrid Fuzzy Differential Equation

P Evangelin Diana Rajakumari^1*, R Gethsi Sharmila²

¹Assistant Professor, PG and Research Department of Mathematics, Bishop Heber College, (Affiliated to Bharathidasan University), Tiruchirappalli-17, Tamil Nadu, India
²Associate Professor, PG and Research Department of Mathematics, Bishop Heber College, (Affiliated to Bharathidasan University), Tiruchirappalli-17, Tamil Nadu, India

*Corresponding Author
Email: [email protected]

Received Date:09 January 2024, Accepted Date:07 April 2024, Published Date:30 April 2024

This work is licensed under a Creative Commons Attribution 4.0 International License.

Abstract

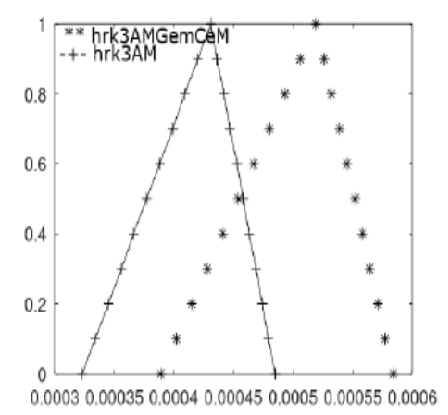
Objectives: To find the absolute error of the Hybrid fuzzy differential equation with triangular fuzzy number since its membership function has a triangular form. Methods: The third order runge-kuttta method based on the linear combination of Arithmetic mean, Geometric mean and Centroidal mean is introduced to solve Hybrid fuzzy differential equation. Seikkala’s derivatives are considered, and Numerical example is given to demonstrate the effectiveness of the proposed method. Findings: The comparative study have been done between the proposed method and the existing third order runge-kutta method based on Arithmetic mean. The proposed method gives better error tolerance than the third order runge-kutta method based on Arithmetic mean. Novelty: In this study a new formula has been developed by combining three means Arithmetic Mean, Geometric Mean and Centroidal Mean using Khattri’s formula and it is solved by the third order runge-kutta method for the first order hybrid fuzzy differential equation. The error tolerance has been calculated for the proposed method and the Arithmetic mean and it is seen that the error tolerance is better for the proposed method.

Keywords: Hybrid fuzzy differential equations, Triangular fuzzy number, Seikkala’s derivative, Third order runge-kutta method, Initial value problem

References

Mukherjee AK, KHG, Salahshour S, Ghosh A, Mondal SP. A Brief Analysis and Interpretation on Arithmetic Operations of Fuzzy Numbers. Results in Control and Optimization. 2023;13:1–42. Available from: https://doi.org/10.1016/j.rico.2023.100312
Rajakumari PED, Sharmila RG. A Third order Runge-Kutta method based on linear combination of Arithmetic mean, Harmonic mean and Heronian mean for hybrid fuzzy differential equation. Journal of Algebraic statistics. 2022;13(2):1049–1057. Available from: https://doi.org/10.52783/jas.v13i2.261
Rajakumari PED, Sharmila RG. A Third order Runge-Kutta method based on linear combination of Arithmetic mean, Heronian mean and Contra Harmonic mean for hybrid fuzzy differential equation. Journal of Algebraic statistics. 2022;13(3):1454–1461. Available from: https://publishoa.com/index.php/journal/article/view/767/658
Sharmila RG, Suvitha S, Sunithy MS. A third order Runge-Kutta method based on a linear combination of arithmetic mean, geometric mean and centroidal mean for first order differential equation. In: PROCEEDINGS OF INTERNATIONAL CONFERENCE ON ADVANCES IN MATERIALS RESEARCH (ICAMR - 2019), AIP Conference Proceedings. (Vol. 2261, Issue 1, pp. 42-46) AIP Publishing. 2020.
Jeyaraj T, Rajan D. Explicit Runge Kutta method in solving fuzzy Initial Value Problem. Advances and Applications in Mathematical Sciences. 2021;20(4):663–674. Available from: https://www.mililink.com/upload/article/1124438296aams_vol_204_feb_2021_a19_p663-674_t._jeyaraj_and_d._rajan.pdf
Buckley JJ, Feuring T. Fuzzy differential equations. Fuzzy Sets and Systems. 2000;110(1):43–54. Available from: https://dx.doi.org/10.1016/s0165-0114(98)00141-9
Kalaiarasi K, Swathi S. Arithmetic Operations of Trigonal Fuzzy Numbers Using Alpha Cuts: A Flexible Approach to Handling Uncertainty. Indian Journal Of Science And Technology. 2023;16(47):4585–4593. Available from: https://doi.org/10.17485/IJST/v16i47.2654
Kanade GD, Dhaigude RM. Iterative Methods for Solving Ordinary Differential Equations: A Review. International Journal of Creative Research Thoughts (IJCRT). 2022;10(2):266–270. Available from: https://www.ijcrt.org/papers/IJCRTL020078.pdf
Singh P, Gor B, Mukherjee S, Mahata A, Mondal SP. Analysis and interpretation of Malaria disease model in crisp and fuzzy environment. Results in Control and Optimization. 2023;12:1–22. Available from: https://doi.org/10.1016/j.rico.2023.100257
Bharathi DP, Jayakumar T, Vinoth S. Numerical Solutions of Fuzzy Multiple Hybrid Single Retarded Delay Differential Equations. International Journal of Recent Technology and Engineering (IJRTE). 2019;8(3):1946–1949. Available from: https://www.ijrte.org/wp-content/uploads/papers/v8i3/C4479098319.pdf
Bharathi DP, TJ, Vinoth S. Numerical Solutions of Fuzzy Pure Multiple Neutral Delay Differential Equations. International Journal of Advanced Scientific Research and Management. 2019;4(1):172–178. Available from: https://ijasrm.com/wp-content/uploads/2019/01/IJASRM_V4S1_1133_172_178.pdf
Sahu R, Sharma AK. Understanding the Unique Properties of Fuzzy concept in Binary Trees. Indian Journal of Science and Technology. 2023;16(33):2631–2636. Available from: https://doi.org/10.17485/IJST/v16i33.874
Singh P, Gazi KH, Rahaman M, Salahshour S, Mondal SP. A Fuzzy Fractional Power Series Approximation and Taylor Expansion for Solving Fuzzy Fractional Differential Equation. Decision Analytics Journal. 2024;10:1–16. Available from: https://dx.doi.org/10.1016/j.dajour.2024.100402
Srinivasan R, Karthikeyan N, Jayaraja A. A Proposed Technique to Resolve Transportation Problem by Trapezoidal Fuzzy Numbers. Indian Journal of Science and Technology. 2021;14(20):1642–1646. Available from: https://dx.doi.org/10.17485/ijst/v14i20.645
Tude S, Gazi KH, Rahaman M, Mondal SP, Chatterjee B, Alam S. Type-2 fuzzy differential inclusion for solving type-2 fuzzy differential equation. Annals of Fuzzy Mathematics and Informatics. 2023;25(1):33–53. Available from: http://www.afmi.or.kr/papers/2023/Vol-25_No-01/PDF/AFMI-25.1(33-53)-H-220122R2.pdf

Copyright

© 2024 Rajakumari & Sharmila. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited. Published By Indian Society for Education and Environment (iSee)