Indian Journal of Science and Technology

Abstract

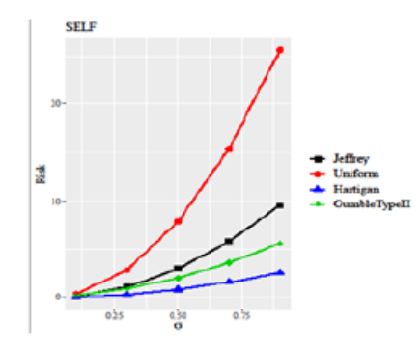
Objectives: To develop Bayesian estimators of dynamic weighted cumulative residual entropy (DWCRE) for Laplace distribution and to investigate posterior risks using various priors and loss functions. Methods: Weighted entropy measure of information is provided by a probabilistic experiment whose basic events are described by their objective probabilities and some qualitative (objective or subjective) weights. In this paper, we have used priors (Jeffrey’s, Hartigan, Uniform and Gumble Type II) and several loss functions. Findings: Bayesian estimators and associated posterior risks for Laplace distribution have been derived for different priors and loss functions. Monte Carlo Simulation study and graphical analyses have also been presented along with the conclusion. Through the comprehensive simulation study in the paper, it has been observed that Hartigan prior is better than other priors in terms of the posterior risk whereas Uniform prior has always higher posterior risk. Novelty: The introduction of new Bayesian estimators and their posterior risks for dynamic weighted cumulative residual entropy (DWCRE) of Laplace distribution.

Keywords: Bayesian estimators, Laplace distribution, Fisher information matrix, Loss functions, Priors