Glued Hypertree: Comparative Analysis and Distance-Based Topological Descriptors

D Antony Xavier; Eddith Sarah Varghese; Deepa Mathew

doi:10.17485/IJST/v15i15.37

Article

Glued Hypertree: Comparative Analysis and Distance-Based Topological Descriptors

VIEWS 1357
PDF 188

Indian Journal of Science and Technology

DOI: 10.17485/IJST/v15i15.37

Year: 2022, Volume: 15, Issue: 15, Pages: 658-667

Original Article

Glued Hypertree: Comparative Analysis and Distance-Based Topological Descriptors

D Antony Xavier¹, Eddith Sarah Varghese^1*, Deepa Mathew²

¹Department of Mathematics, Loyola College, Affiliated to University of Madras, Chennai, India
²Department of Mathematics, St Joseph’s College, Bangalore, India

*Corresponding Author
Email: [email protected]

Received Date:05 January 2022, Accepted Date:05 March 2022, Published Date:14 April 2022

This work is licensed under a Creative Commons Attribution 4.0 International License.

Abstract

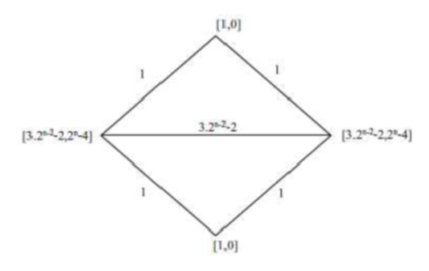
Objectives : To introduce a new interconnection network, Glued hypertree, and to discuss and analyze its physicochemical properties using distance-based topological descriptors. A comparative analysis between the Glued tree and Glued hypertree is carried away in this paper. Methods: We compare glued hypertree with glued tree using some topological parameters. The approach to finding the topological indices is to partition the edge set using Djokovic Wrinkler relation and thus reduce it to quotient graphs. Findings: Distancebased topological indices of Glued hypertree were calculated and also we have analyzed how glued hypertree is better than glued tree. Novelty: We have evaluated and compared the various topological indices of Glued hypertree using a graphical representation.

Keywords: Glued Hypertree; Distance-based Indices; Messages Traffic Density; Average Distance

References

Xu J. Topological Structure and Analysis of Interconnection Networks. Book. Springer US. 2013.
Rajan RS, Kumar KJ, Shantrinal AA, Rajalaxmi TM, Rajasingh I, Balasubramanian K. Biochemical and phylogenetic networks-I: hypertrees and corona products. Journal of Mathematical Chemistry. 2021;59(3):676–698. Available from: https://dx.doi.org/10.1007/s10910-020-01194-3
Childs AM, Farhi E, Gutmann S. An example of the difference between quantum and classical random walks. Quantum Information Processing. 2002;1:35–43. Available from: https://dx.doi.org/10.1023/a:1019609420309
Childs AM, Cleve R, Deotto E, Farhi E, Gutmann S, Spielman DA. Exponential algorithmic speedup by a quantum walk. Proceedings of the thirty-fifth ACM symposium on Theory of computing - STOC '03. 2003;p. 59–68. Available from: https://doi.org/10.1145/780542.780552
Rani LN, Rajkumari KJ, Roy S. Wiener Index of Hypertree. In: Trends in Mathematics. (pp. 497-505) Springer International Publishing. 2019. 10.1007/978-3-030-01123-9_49
Balasubramanian K. Computational and Artificial Intelligence Techniques for Drug Discovery and Administration. Reference Module in Biomedical Sciences. 2021. Available from: https://doi.org/10.1016/B978-0-12-820472-6.00015-3
Balasubramanian K. Combinatorics, Big Data, Neural Network & AI for Medicinal Chemistry & Drug Administration. Letters in Drug Design & Discovery. 2021;18(10):943–948. doi: 10.2174/1570180818666210719130052
Nandini GK, Rajan RS, Shantrinal AA, Rajalaxmi TM, Rajasingh I, Balasubramanian K. Topological and Thermodynamic Entropy Measures for COVID-19 Pandemic through Graph Theory. Symmetry. 1992;12(12):1992. Available from: https://doi.org/10.3390/sym12121992
Mondal S, De N, Pal A. Topological Indices of Some Chemical Structures Applied for the Treatment of COVID-19 Patients. Polycyclic Aromatic Compounds. 2020;p. 1–15. Available from: https://dx.doi.org/10.1080/10406638.2020.1770306
Klavzar S, Gutman I, Mohar B. Labeling of Benzenoid Systems which Reflects the Vertex-Distance Relations. Journal of Chemical Information and Computer Sciences. 1995;35(3):590–593. Available from: https://dx.doi.org/10.1021/ci00025a030
Klavzar S, Nadjafi-Arani M. Cut Method: Update on Recent Developments and Equivalence of Independent Approaches. Current Organic Chemistry. 2015;19(4):348–358. Available from: https://dx.doi.org/10.2174/1385272819666141216232659
Arockiaraj M, Clement J, Balasubramanian K. Topological Indices and Their Applications to Circumcised Donut Benzenoid Systems, Kekulenes and Drugs. Polycyclic Aromatic Compounds. 2020;40(2):280–303. Available from: https://doi.org/10.1080/10406638.2017.1411958
Arockiaraj M, Clement J, Tratnik N, Mushtaq S, Balasubramanian K. Weighted Mostar indices as measures of molecular peripheral shapes with applications to graphene, graphyne and graphdiyne nanoribbons. SAR and QSAR in Environmental Research. 2020;31(3):187–208. Available from: https://dx.doi.org/10.1080/1062936x.2019.1708459
Klavžar S, Manuel P, Nadjafi-Arani MJ, Rajan RS, Grigorious C, Stephen S. Average Distance in Interconnection Networks via Reduction Theorems for Vertex-Weighted Graphs. The Computer Journal. 2016;59(12):1900–1910. Available from: https://dx.doi.org/10.1093/comjnl/bxw046
Klavžar S, Nadjafi-Arani MJ. Wiener index in weighted graphs via unification ofΘ∗-classes. European Journal of Combinatorics. 2014;36:71–76. Available from: https://dx.doi.org/10.1016/j.ejc.2013.04.008
Imran M, Siddiqui MK, Baig AQ, Shaker H. Molecular topological description of bacterial hypertrees. Journal of Intelligent & Fuzzy Systems. 2020;38(4):5095–5105. Available from: https://dx.doi.org/10.3233/jifs-191714

Copyright

© 2022 Xavier et al. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited.

Published By Indian Society for Education and Environment (iSee)